Welcome to the realm of Unit 4 Solving Quadratic Equations Answer Key, where the intricacies of quadratic equations unravel, revealing their significance in the tapestry of mathematics. This comprehensive guide will illuminate the concepts, methods, and applications of quadratic equations, equipping you with the knowledge to conquer these equations with confidence.
Through a systematic exploration of factoring, completing the square, and the quadratic formula, this guide will empower you to tackle quadratic equations with precision and efficiency. Delve into the practical applications of these equations in diverse fields, unraveling their relevance in the real world.
1. Understanding Quadratic Equations: Unit 4 Solving Quadratic Equations Answer Key
Quadratic equations are mathematical equations of the form ax^2 + bx + c = 0, where a, b, and c are constants and x is the variable. They are called quadratic because the highest power of x is 2.
Key Components of a Quadratic Equation
- a: The coefficient of x^2, which determines the curvature of the parabola.
- b: The coefficient of x, which determines the axis of symmetry of the parabola.
- c: The constant term, which determines the y-intercept of the parabola.
2. Solving Quadratic Equations
Factoring
Factoring involves expressing the quadratic equation as a product of two linear factors. This method works well when the equation can be easily factored into two binomials.
Completing the Square
Completing the square involves adding and subtracting a constant to the equation to create a perfect square trinomial. This method is useful when factoring is not possible.
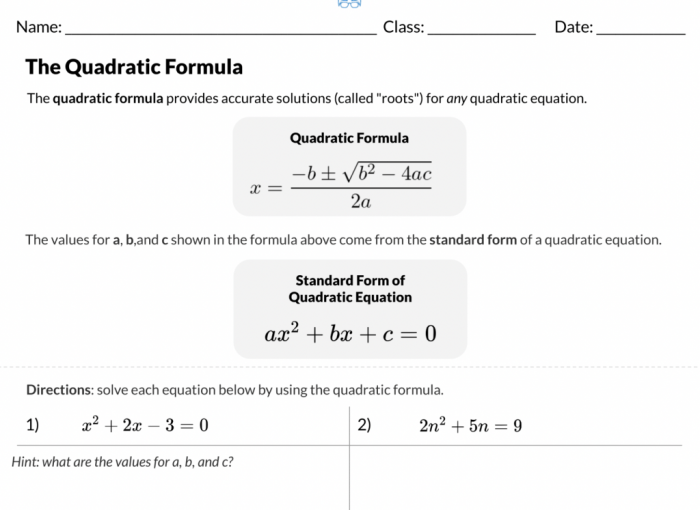
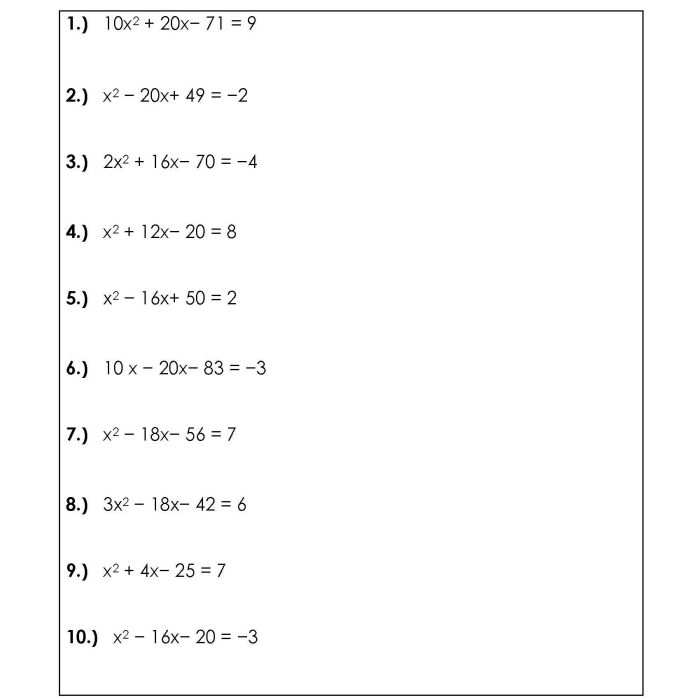
Quadratic Formula
The quadratic formula is a general method that can be used to solve any quadratic equation. It involves using the formula (-b ± √(b^2 – 4ac)) / 2a.
3. Factoring Quadratic Equations

Steps for Factoring
- Set the equation equal to zero.
- Factor out any common factors.
- Use the difference of squares, perfect square trinomials, or other factoring techniques to factor the remaining quadratic.
Special Cases
- Difference of Squares: (a + b)(a – b)
- Perfect Square Trinomials: (a + b)^2, (a – b)^2
4. Completing the Square
Steps for Completing the Square
- Move the constant term to the other side of the equation.
- Divide both sides by the coefficient of x^2.
- Add the square of half the coefficient of x to both sides.
- Factor the left side as a perfect square trinomial.
5. Quadratic Formula
Using the Quadratic Formula
To use the quadratic formula, simply plug the values of a, b, and c into the formula and solve for x.
Discriminant, Unit 4 solving quadratic equations answer key
The discriminant, b^2 – 4ac, determines the number of solutions to a quadratic equation:
- Positive Discriminant: Two real solutions
- Zero Discriminant: One real solution (a double root)
- Negative Discriminant: No real solutions (complex solutions)
6. Applications of Quadratic Equations
Real-World Applications
Quadratic equations have numerous applications in various fields, including:
- Physics: Projectile motion, parabolic trajectories
- Engineering: Structural analysis, fluid dynamics
- Finance: Modeling investment returns, loan payments
Question Bank
What is the standard form of a quadratic equation?
The standard form of a quadratic equation is ax^2 + bx + c = 0, where a, b, and c are real numbers and a is not equal to 0.
How do I factor a quadratic equation?
To factor a quadratic equation, you can use a variety of methods, including trial and error, grouping, and the quadratic formula.
What is the quadratic formula?
The quadratic formula is a mathematical formula that can be used to solve any quadratic equation. The formula is: x = (-b ± √(b^2 – 4ac)) / 2a, where a, b, and c are the coefficients of the quadratic equation.